
Only the bottom of the box of is cut by the electric flux. The sides of the box are parallel to the electric field and hence no electric flux cuts these. The top of the box is within the capacitor plate and does not have any electric flux cutting it. To calculate the electric field flux we introduce a suitable Gaussian surface (a rectangular box in this case). Note: The net charge outside any capacitor is always zero. In reality, the electric field at the edges of the plates would not be a straight line but would slightly extend beyond the capacitor geometry. Note: For calculation we assume a uniform electrical field. This creates an electrical field between the plates. Two plates of area A and separated by a distance d are charged ( +Q on one plate and -Q on the other plate). The illustration shows a parallel plate capacitor. For illustration consider the case of a parallel plate capacitor. Gauss's Law can be used to find the capacitance of any arrangement. The ratio of charge on the plates to the voltage between the plates is a constant and is the capacitance: The stronger the electric field, the more lines are drawn to represent the field.Ī capacitor consists of two surfaces (one positively charged and one negatively charged). The lines are taken to travel from positive charge to negative charge. The magnitude of an electric field is expressed in newtons per coulomb, which is equivalent to volts per metre.Įlectric fields are often represented by the concept of field lines.

An electric field is a vector acting in the direction of any force on a charged particle.
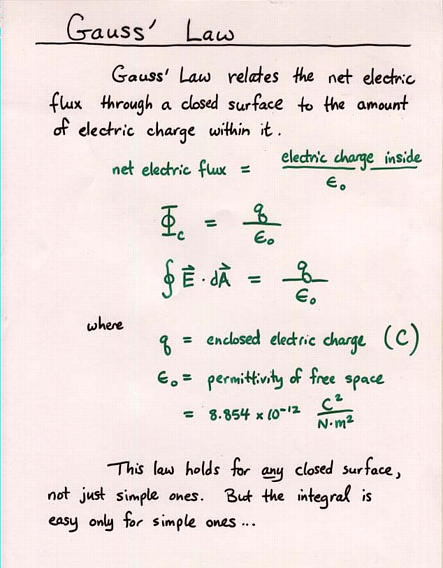
In a physical sense, it describes the force which would be exerted on a charged particle within the field. Electric Field and Electric FluxĮlectric fields are generated by charged particles (and varying magnetic fields). Conversely knowing the charge contained within a surface, the electric flux passing through the surface can be determined. Together both these terms define the law in mathematical concepts.īy knowing an electric flux distribution through a surface, we can find the charge contained by the surface. The second term is the net charge ( q in Coulombs) enclosed within the surface (divided by a constant of proportionality 1/ε0). The dot product gives the electrical field normal to the surface and the integral the total electric flux ( ) passing through the surface. The first term tells us to take the surface integral of the dot product between electric vector ( E in V/m) and a unit vector ( n) normal to the surface. Gauss's Electric Field Law - Integral Form In integral form, we write Gauss's Electric Field Law as: The post is relatively short, but it does give an overview of Maxwell's Equations and puts them into context. The electric field flux passing through a closed surface is proportional to the charged contained within that surfaceĪt this stage, if you have not read our Maxwell's Equations Introduction post it is worth reading. More formally it relates the electric flux passing through a closed surface to the charge contained within the surface. The law shows how the electrostatic field behaves and varies depending on the charge distribution within it. In Gauss's law, the electric field is the electrostatic field. The law was initially formulated by Carl Friedrich Gauss in 1835.

Gauss's Electrical law defines the relation between charge ("Positive" & "Negative") and the electric field.


 0 kommentar(er)
0 kommentar(er)
